题库 / Prep2007E1-DS-181
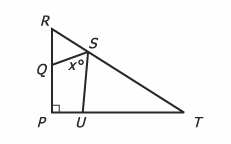
In the figure shown, what is the value of x ?
(1) The length of line segment QR is equal to the length of line segment RS.
(2) The length of line segment ST is equal to the length of line segment TU.
Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
EACH statement ALONE is sufficient.
Statements (1) and (2) TOGETHER are NOT sufficient.
还没有题目讲解(毕出老师会陆续发布对官方考题的解读,请保持关注)。
求X的度数? (设X左侧的角叫S1, X右侧的角叫S2,则X+S1+S2=180平角)
1-条件一知三角形QRS是等腰三角形--->推出R+2S1=180;不能得出X,不充分;
2-条件二知三角形SUT是等腰三角形---->推出T+2S2=180;不能得出X,不充分;
(1)+(2)联立,加题干已知P直角 :
R+2S1=180
T+2S2=180
R+T=90
可推出S1+S2,同时X+S1+S2=180,即可得出X
无法加载图片
求X的度数? (设X左侧的角叫S1, X右侧的角叫S2,则X+S1+S2=180平角)
1-条件一知三角形QRS是等腰三角形--->推出R+2S1=180;不能得出X,不充分;
2-条件二知三角形SUT是等腰三角形---->推出T+2S2=180;不能得出X,不充分;
(1)+(2)联立,加题干已知P直角 :
R+2S1=180
T+2S2=180
R+T=90
可推出S1+S2,同时X+S1+S2=180,即可得出X
赞
登录 或 注册 后可以参加讨论
求X的度数?
(1)QR=RS 只知道rqs这个三角形是等腰三角形;不充分
(2)ST=TU 只知道tsu这个三角形是等腰三角形;不充分
联立:Lrqs=Lrsq
Lsut=Lust
180=Lrsq+X+Lust=Lrqs+Lsut+x(1)
sqp+x+90+sup=360
又因为:180-sut=sup
180-rqs=sqp
所以180-rqs+x+90+180-sut=360(2)
联立1 2:x=45度
充分