题库 / GMATLA-MSR-10
Kilometer Run
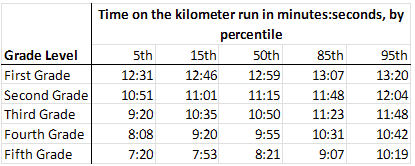
The Huffingdale All Boys School has produced a comprehensive set of running standards for young boys. These standards are based on data from young boys attending Huffingdale in good health conditions - as determined by a physician. The table displays the percentile distribution of time on a kilometer run for Huffingdale students at grade levels 1 through 5 according to the Huffingdale model. In a model population -- a large population of young students grade 1 through 5 that conforms to the Huffingdale running standards -- for n=5, 15, 50, 85, and 95, the nth percentile in running time for a given grade is the unique running time among boys of that grade level that is slower than or equal to n percent, and faster than or equal to (100 - n) percent, of running times for students of that grade level.
Height
The graph shows the percentile distribution of height, in centimeters, for running times from 500 to 700 seconds, according to the Huffingdale model. In a model population, for n=5, 15, 50, 85, and 95, the nth percentile in height for a given running time is the unique height among boys of that running time that is taller than or equal to n percent, and shorter than or equal to (100-n) percent, of heights of boys of that running time.
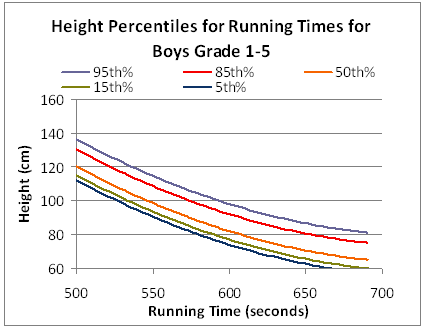
Consider an individual boy from a model population. Suppose that from grades 1 through 5, this boy's height is at the 50th percentile for his running time and his running time is at the 50th percentile for his grade level. Which one of the following statements must be true of the boy at Grade 5?
His grade level is at the 50th percentile for his running time.
His height is at the 50th percentile for his grade level.
His running time is at the 50th percentile for his height.
His height is approximately 130% of his height in Grade 2.
His height is approximately 185% of his height in Grade 2.
E,since his running time at grade 5 is 501 seconds and his height would be 120 cm while at grade 2 it would be 65 cm ,which is 185% of the height at grade 5
登录 或 注册 后可以参加讨论